decide what number must be added to each binomial to make a perfect square trinomial.
18
THE Square OF A BINOMIAL
Perfect square trinomials
The square numbers
The foursquare of a binomial
Geometrical algebra
2d level
(a + b)³
The square of a trinomial
Completing the foursquare
LET US Begin past learning about the square numbers. They are the numbers
1·one 2·two 3·3
and so on. The following are the outset ten foursquare numbers -- and their roots.
| Square numbers | 1 | four | 9 | 16 | 25 | 36 | 49 | 64 | 81 | 100 |
| Square roots | 1 | ii | 3 | iv | 5 | 6 | 7 | 8 | ix | 10 |
1 is the square of 1. four is the square of 2. 9 is the square of 3. And so on.
The square root of i is 1. The square root of 4 is 2. The square root of 9 is 3. And so on.
In a multiplication table, the square numbers lie forth the diagonal.
The square of a binomial
(a + b)2
The square of a binomial comes up then oft that the pupil should be able to write the final product immediately. It will turn out to be a very specific trinomial. To see that, let us square (a +b):
(a + b)2 = ( a + b )( a + b ) = a two + 2ab + b ii.
For, the outers plus the inners will be
ab + ba = twoab.
The square of whatsoever binomial produces the following trinomial:
(a + b)two = a 2 + twoab + b 2
These will be the three terms:
1. The square of the first term of the binomial:a 2
2. Twice the production of the two terms: 2ab
3. The foursquare of the second term:b two
The square of a binomial is a essential form in the "multiplication table" of algebra.
See Lesson viii of Arithmetics: How to foursquare a number mentally, specially the square of 24, which is the "binomial" twenty + four.
Example ane. Square the binomial (x + 6).
Solution. (ten + 6)ii = x ii + 12x + 36
ten 2 is the square of x.
12x istwice the product of ten with 6. (10 · six = 6x. Twice that is 1210.)
36 is the square of half dozen.
The square of a binomial is chosen a perfect square trinomial.
x ii + 12x + 36 is a perfect square trinomial.
Instance two. Square the binomial (threex − 4).
Solution. (3x − 4)2 = 9x 2 − 24ten + xvi
9x 2 is the square of threex.
−24x istwice the product of 3ten · −4. (3x · −4 = −12x. Twice that is −24x.)
16 is the square of −four.
Note: If the binomial has a minus sign, and then the minus sign appears only in the middle term of the trinomial. Therefore, using the double sign ± ("plus or minus"), we can country the rule equally follows:
(a ± b)2 = a 2 ± twoab + b 2
This means: If the binomial is a + b, then the middle term volition be +2ab; but if the binomial is a − b, and so the middle term will be −2ab
The square of +b or −b, of class, is always positive. Information technology is always +b 2.
Example 3. (5ten 3 − 1)2 = 2510 half-dozen − 10ten 3 + 1
25x vi is the foursquare of 5x three. (Lesson 13: Exponents.)
−10x 3 istwice the production of vx iii and −one. (vx three times −ane = −5x 3. Twice that is −10x 3.)
1 is the square of −1.
The educatee should exist clear that (a + b)two is not a ii + b two, any more (a + b)3 is equal to a 3 + b 3.
An exponent may not be "distributed" over a sum.
(See Topic 25 of Precalculus: The binomial theorem.)
Trouble one.
a) State in words the rule for squaring a binomial.
The square of the first term.
Twice the production of the two terms.
The square of the 2nd term.
b) Write only the trinomial production: (x + 8)2 = x 2 + 1610 + 64
c) Write only the trinomial product: (r +due south)2 = r 2 + 2rs + s 2
Problem 2. Write simply the trinomial product.
| a) | (10 + ane)two = ten 2 + 2ten + 1 | b) (x − 1)2 = | x 2 − 2x + 1 | |
| c) | (x + 2)2 = x ii + 4x + iv | d) (x − 3)two = | ten two − half-dozenx + 9 | |
| due east) | (10 + iv)2 = ten 2 + 8x + 16 | f) (x − 5)2 = | x two − 10x + 25 | |
| yard) | (x + 6)2 = x 2 + 12x + 36 | h) (x − y)2 = | 10 ii − 2xy + y ii | |
Trouble 3. Write only the trinomial production.
| a) | (2ten + 1)two =4ten two + 4x + 1 | b) (3x − 2)2 = | 9x 2 − 12x + iv | |
| c) | (fourten + iii)2 =16x 2 + 24x + 9 | d) (5x − ii)2 = | 25x two − xxten + four | |
| e) | (x iii + i)2 = ten vi + twox 3 + 1 | f) (x 4 − three)2 = | x 8 − 6x 4 + 9 | |
| g) | (x n + 1)2 = 10 2n + 2x n + one | h) (10 n − 4)two = | x twon − 8x northward + 16 | |
Example 4. Is this a perfect foursquare trinomial:ten 2 + 14x + 49 ?
Answer. Yes. It is the foursquare of (x + seven).
x 2 is the foursquare of ten. 49 is the foursquare of 7. And 14x is twice the product of x · 7.
In other words, x 2 + 1410 + 49 could exist factored as
ten ii + 14x + 49 = (x + vii)ii
Note: If the coefficient of x had been whatsoever number merely xiv, this would not accept been a perfect square trinomial.
Instance 5 Is this a perfect square trinomial:10 2 + fiftyx + 100 ?
Respond. No, it is non. Although 10 ii is the square of x, and 100 is the square of 10, 50ten is not twice the product of x · 10. (Twice their production is 20x.)
Case six Is this a perfect foursquare trinomial:x viii − 16x 4 + 64 ?
Reply. Yes. It is the perfect square often iv − 8.
Problem iv. Cistron:p 2 + iipq + q 2.
p ii + 2pq + q 2 = (p + q)two
The left-hand side is a perfect square trinomial.
Problem 5. Factor as a perfect square trinomial -- if possible.
| a) | x ii − 410 + 4= (10 − 2)2 | b) | x ii + 610 + ix= (x + three)two | |
| c) | 10 ii − xviiix + 36 Not possible. | d) | x two − 1210 + 36= (x − 6)2 | |
| e) | x 2 − three10 + 9 Not possible. | f) | x 2 + 10x + 25= (x + five)2 | |
Trouble half-dozen. Gene as a perfect square trinomial, if possible.
a) 25x two + 30x + 9= (5x + 3)2
b) 4ten 2 − 28x + 49= (2x − 7)2
c) 25x 2 − 10x + 4 Non possible.
d) 25x 2 − 2010 + 4= (vx − ii)2
east) ane − 16y + 64y2= (ane − 8y )2
f) 161000 2 − xlmn+ 25n 2= (4m − vn)ii
thou) 10 four + 2x 2 y 2 + y four = (x 2 + y 2)2
h) ivx six − 1010 3 y iv + 25y 8 Not possible.
i) x 12 + viiix 6 + xvi = (x 6 + 4)2
j) x 2n + viiix n + 16 = (ten due north + 4)2
Geometrical algebra
Hither is a square whose side is a + b.
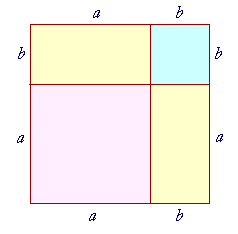
It is composed of
a square whose side is a,
a square whose side is b,
and two rectangles ab.
That is,
(a + b)two = a 2 + 2ab + b 2.
second Level
Next Lesson: The difference of two squares
Table of Contents | Dwelling
Please make a donation to go along TheMathPage online.
Even $ane will help.
Copyright © 2021 Lawrence Spector
Questions or comments?
Email: teacher@themathpage.com
Source: https://themathpage.com/Alg/perfect-square-trinomial.htm
Belum ada Komentar untuk "decide what number must be added to each binomial to make a perfect square trinomial."
Posting Komentar